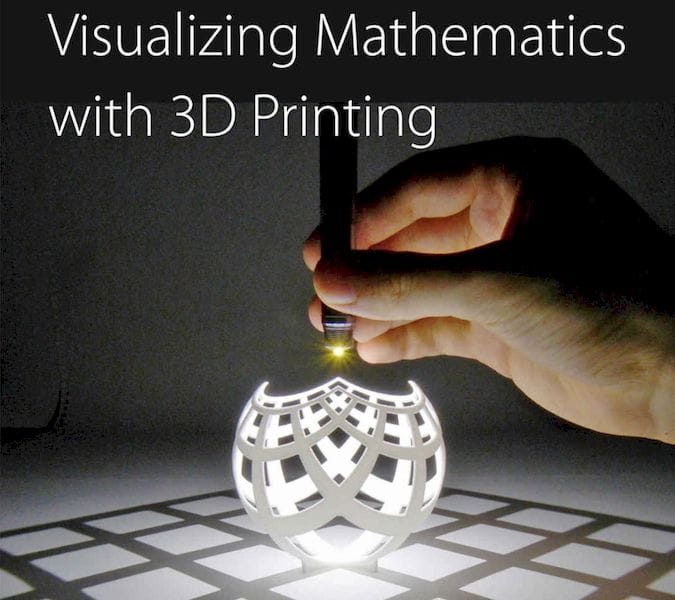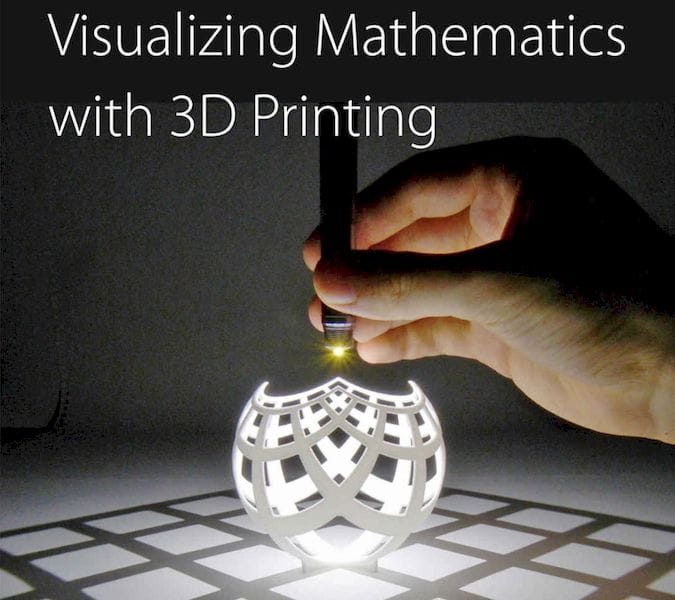
This week’s selection is the fascinating “Visualizing Mathematics with 3D Printing” by Henry Segerman.
Segerman is a mathematician and artist, and those two disciplines are definitely present in this startling book that explores the nature of highly complex mathematically generated shapes.
The problem is that such shapes are often very difficult to understand; their shapes are so complex you cannot properly represent them with a 2D image (of which there are many in this book).
To aid the reader, Segerman operates a companion web site in which you can load up 3D models of the specific mathematical shapes and then twirl them around in a 3D view.
However, the best approach is to physically produce the objects themselves by downloading 3D printable files and printing them.
If so you’ll soon be holding a hyperbolic honeycomb, like this:
Segerman reviews a number of different types of mathematical objects in the book. He explains:
The first chapter is about different ways that three dimensional objects can be symmetric.
Chapter 2 is about some of the simplest shapes: the two-dimensional polygons and the polyhedra, their three-dimensional relatives.
Chapter 3 builds off chapter 2, reaching up to the four-dimensional relatives of polygons and polyhedra and investigating how we can see four-dimensional objets by casting shadows of them down to three dimensions.
Chapter four is about tilings and curvature – whether a surface is shaped like a hill, a flat plane or a saddle.
Chapter 5 is about knots and thinking topologically – looking at geometric objects but not caring about the precise shapes, as if everything were made of very stretchy rubber.
Chapter 6 continues the topological theme by looking at surfaces and then later on thinking about geometry again by putting tilings on surfaces.
Chapter 7 is a menagerie, of mathematical prints I couldn’t resist including in the book.
Don’t be afraid if you don’t know much about mathematics; Segerman’s prose is easy to follow and is direct at all the public.
Via Amazon